Unexpected curves: Drawing straight lines on a map

A combination of flight paths, a calculation of the direction of Mecca and sea routes. All about geodesic lines.

One of the things that most often catches the attention of people who are not obsessed with cartography and maps is the representation of the shortest distance between two points. Almost everyone knows that maps distort reality, but when someone asks us to draw the shortest route between two points, our first impulse is to draw a straight line between the two on the map. This approach may work in some cases, depending on the projection used, or when the two points are close together on the map¹, but often the solution will be much more complex than expected.

This is much easier to accept when you see it for yourself. If you have a globe at home and a piece of string, just draw lines and observe that in some cases the paths are not as intuitive as they might seem. These lines are known in geometry as geodesics. Today, I will bring some examples and, from there, I'll explore some curious lines that can be drawn on the planet.

Airline routes

If you have ever taken a transoceanic flight without changing hemispheres, or between Europe and Asia, you have probably noticed that the path followed by aeroplanes is not usually a straight line on the map. When I first crossed the Atlantic for the first time, I experienced this myself for the first time. I had just changed jobs, and I was sent to Boston for a few weeks of training. Due to the schedule and cost, I had to stop over in Munich, so the trip was more or less like the one you can see on the map below.

Route Madrid - Munich - Boston (Google Maps)

I had read and studied about map projections, but when I saw this for the first time, what came to mind was that there were other reasons why this route had been chosen. I assumed that, having to spend so many hours over the ocean, it was simply a matter of safety to decide to leave Europe via the British Isles and enter North America via Newfoundland. It sounded wonderful to me, and in a way, it was the perfect explanation. It's fascinating how we can fool ourselves when we are truly convinced of something.

A few years later, I had another long trip, but this time in the completely opposite direction. I went to Japan to visit a friend, and the best option I could find was flying from Madrid to London, and from there to Tokyo. The route was similar to what you can see on the map below.

Route Madrid - London - Tokyo (Google Maps)

This is where my mental framework collapsed. The simple explanation I had come up with for the trip to Boston no longer worked in this case. We're not talking about a slight detour; in this case, the plane flew slightly over the Arctic Ocean, tracing a curve on the map that was not at all intuitive. It became obvious as soon as I read a bit on the topic. Planes generally take the shortest route between two points, as it reduces fuel cost, but these routes correspond to the geodesics on the Earth's surface, not the straight lines on the map. Taking the map above, the distance between London and Tokyo along the geodesic line is 9,576 kilometres². If, on the other hand, we draw a straight line on the map and measure the distance, we get more than 11,200³ kilometres, which is an increase of 17%. Almost no difference, right?

---

In the early years of commercial aviation, it was common for airlines to advertise with maps. This would allow potential customers to appreciate the great distances that could be covered thanks to the new technological advance. As you'd expect, those early maps showed the limitations of the technology of the time: planes could only fly a minimum distance before stopping to refuel, which meant multiple stopovers. To take advantage of the situation, airlines flew over populated areas and places of interest, so more people could benefit from the overall route. This is why some maps draw almost straight lines across the map along the entire route, as in this example from the Dutch airline KLM.

KLM flight map from 1929 (source)

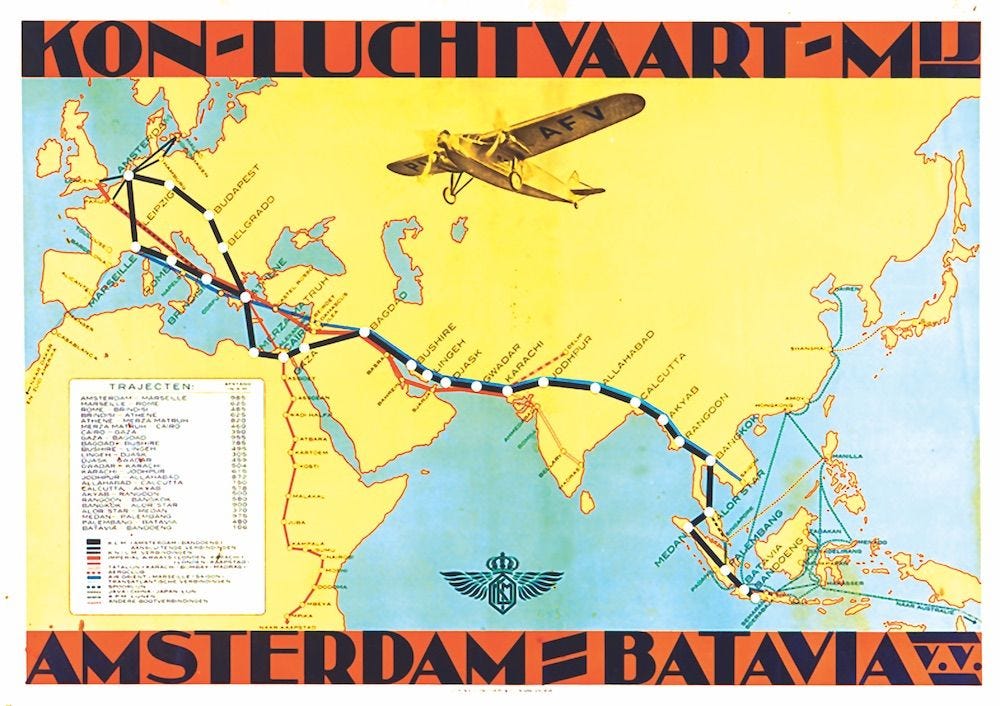
As aircraft became more autonomous and safer, routes became more efficient, which was also reflected in the airline route maps published by airlines. Perhaps the most iconic maps are those published by SAS (Scandinavian Airlines Systems) during the 1960s and 1970s. Using unusual projections, they effectively showed how aircraft could make journeys that were impossible for other means of transport. The map below clearly shows a flight between Copenhagen and Alaska that crosses the Arctic Ocean directly.

Scandinavian Airlines System, 1960 (source)

Facing Mecca

According to Islam, all daily prayers must be performed facing the city of Mecca, where the Kaaba is located. This also determines the orientation of mosques, as these buildings are constructed in such a way that prayers inside them are also facing Mecca⁴.

The Islamic Centre of Washington was built between 1949 and 1953 and, following Muslim tradition, was constructed with the prayer hall⁵ facing Mecca. Shortly before its opening, the building was visited by Kamil Abdul Rahim, ambassador of the Arab League to the United Nations, who questioned whether the orientation of the building was correct. Mecca is located east-southeast of Washington, but the building pointed northwest.

Orientation of the Washington Islamic Centre (source)

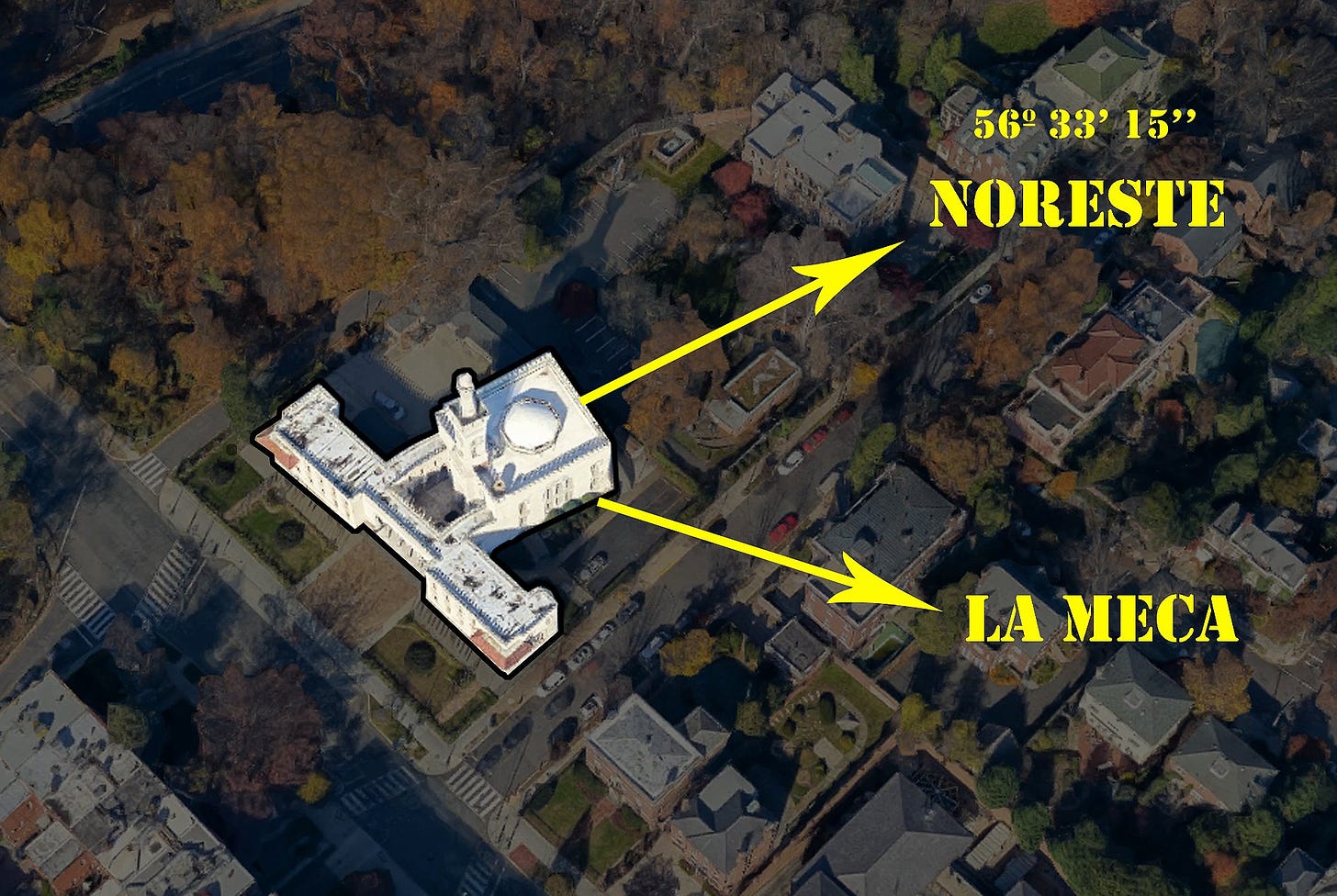
And here we find ourselves again with the same dilemma we have been discussing with the airlines. The geodesic line connecting Washington and Mecca corresponds to the orientation of the mosque (which you can see on the map below in black), while the straight line that can be drawn on the map corresponds to the direction that Kamil Abdul Rahim interpreted as correct (in orange on the map below). In a way, depending on how you interpret it, it appears that both options might be correct.

Washington - Mecca (Google Maps)

Which one does Islam consider correct?

Centuries ago, when there was no access to any tools to establish where Mecca was located, it was common for each region to have markers in its local mosque. In general, to the west of Mecca, it was recommended to pray towards the sunrise, while to the east of Mecca, towards the sunset. With the popularisation of compasses, this idea was perpetuated as the most practical way for any Muslim to orient themselves approximately towards Mecca.

The reality is that Muslim mathematicians solved this dilemma several centuries ago. According to them, the best way is to orient yourself along the geodesic, along the shortest distance between you and Mecca. As you might expect, Islam is flexible enough to accept any approximation as good.

Back to the Washington building, the architect was right, not so much the ambassador.

The longest sea routes

You may have heard that one of the great things about the Mercator projection is its effectiveness for navigation. By its mathematical definition, it is a conformal projection, which allows it to maintain angles. In practice, this means that is that any straight line drawn on a map with this projection can be easily followed by a sailor as long as they maintain a constant course⁶. This was common practice during the 17th and 18th centuries, when much of the world's oceans were explored. But a peculiarity of the course is that it does not help to travel the shortest distance; it is simply the simplest and most intuitive way to get from A to B.

The big difference between course and geodesic is often quite interesting to explore. With the popularisation of Google Earth and Google Maps, many people have spent part of their time plotting navigation routes along geodesic lines, so that unexpected points can be connected. In a way, this is equivalent to thinking: if I stand on the coast and look towards the horizon, where am I looking to?

Surprisingly, there is a point on the coast of Norway, near Bergen, that points directly towards Antarctica, but across the Arctic Ocean!

Route between Norway and Antarctica (source)

Along the same lines, Patrick Anderson suggested in 2012 on Reddit that he had found the longest geodesic line that could be travelled over the sea without stepping on land: from the coast of Pakistan to the Kamchatka Peninsula in Russia. If you want something more visually appealing, you can check out this YouTube video where he traced the same line on Google Earth, but below is the map he originally posted on Reddit.

From Pakistan to Kamchatka (source)

Rohan Chabukswar and Kushal Mukherjee found this idea fascinating enough to dedicate time to formalize and validate it, or find the longest route that can actually be travelled in a straight line. After five years of brute force analysis, in which more than 230 million possibilities were analysed, in 2018 they published that Patrick Anderson was correct⁷.

Specifically, the sea route would start from a beach near Sonmiani, in Pakistan. It would follow the east coast of Africa, cross the Mozambique Channel, which separates Madagascar from the continent, and reach the Antarctic Ocean. From there, it would pass between Cape Horn and Antarctica, cross the entire Pacific Ocean and finally reach the cold beaches of Karaginsky Island, off the coast of Kamchatka, Russia.

With all the data in hand, the authors of this study also revealed what the equivalent route by land would be, going from Sagres, in southern Portugal, to the coast of China, near the city of Jinjiang⁸. I'll leave this below as a bonus for today's article.

The world's longest straight line route by land (source)

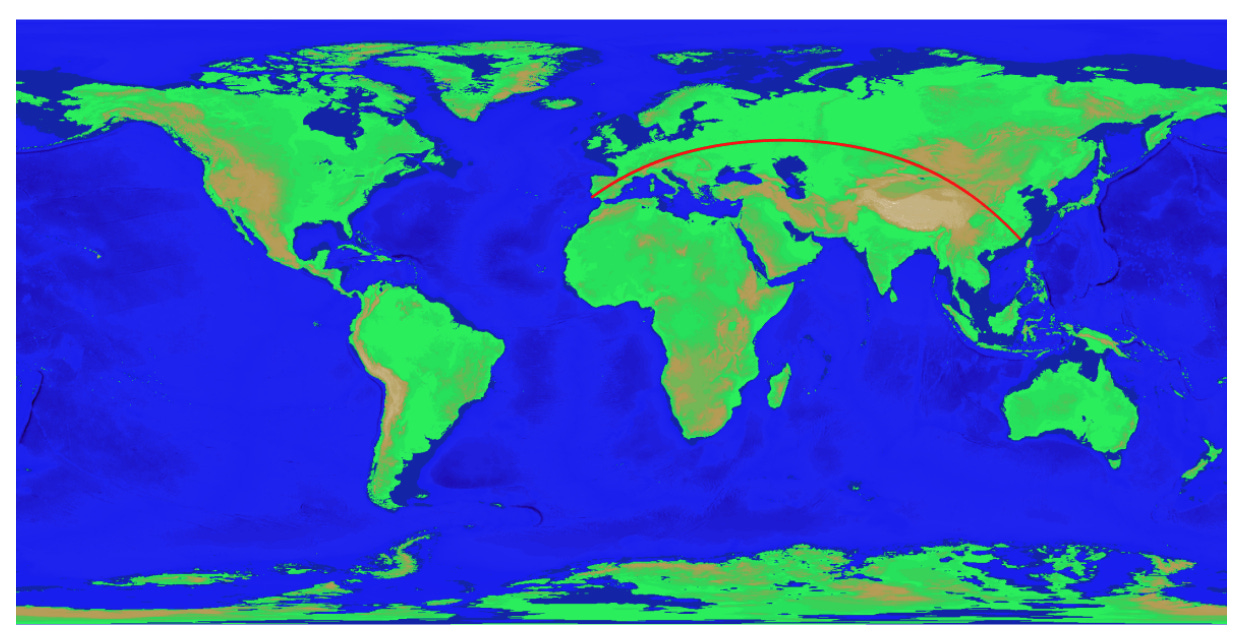
1. It is not possible to generalise, as the distortion will vary depending on the orientation of the line and the projection. But if we take a horizontal line with the projection used by Google Maps, it takes more than 1,000 kilometres (621 miles) to observe the curve.

2. 5,950 miles.

3. 6,959 miles.

4. This direction is named qibla.

5. Also known as muṣallá.

6. The bearing, by mathematical definition, is the angle between the line from a point to the north and the direction you are going to take. In more practical terms, maintaining the course simply means ensuring that you are navigating with the compass showing the same bearing, always pointing in the same direction.

7. Here are the details of the study in Science magazine. If you would like the full paper, you can download it in PDF format here.

8. You may have seen another similar route that runs from the Pacific coast of Asia to the Atlantic coast of Africa. This other route crosses the Dead Sea, so it is not usually considered correct.